Projects

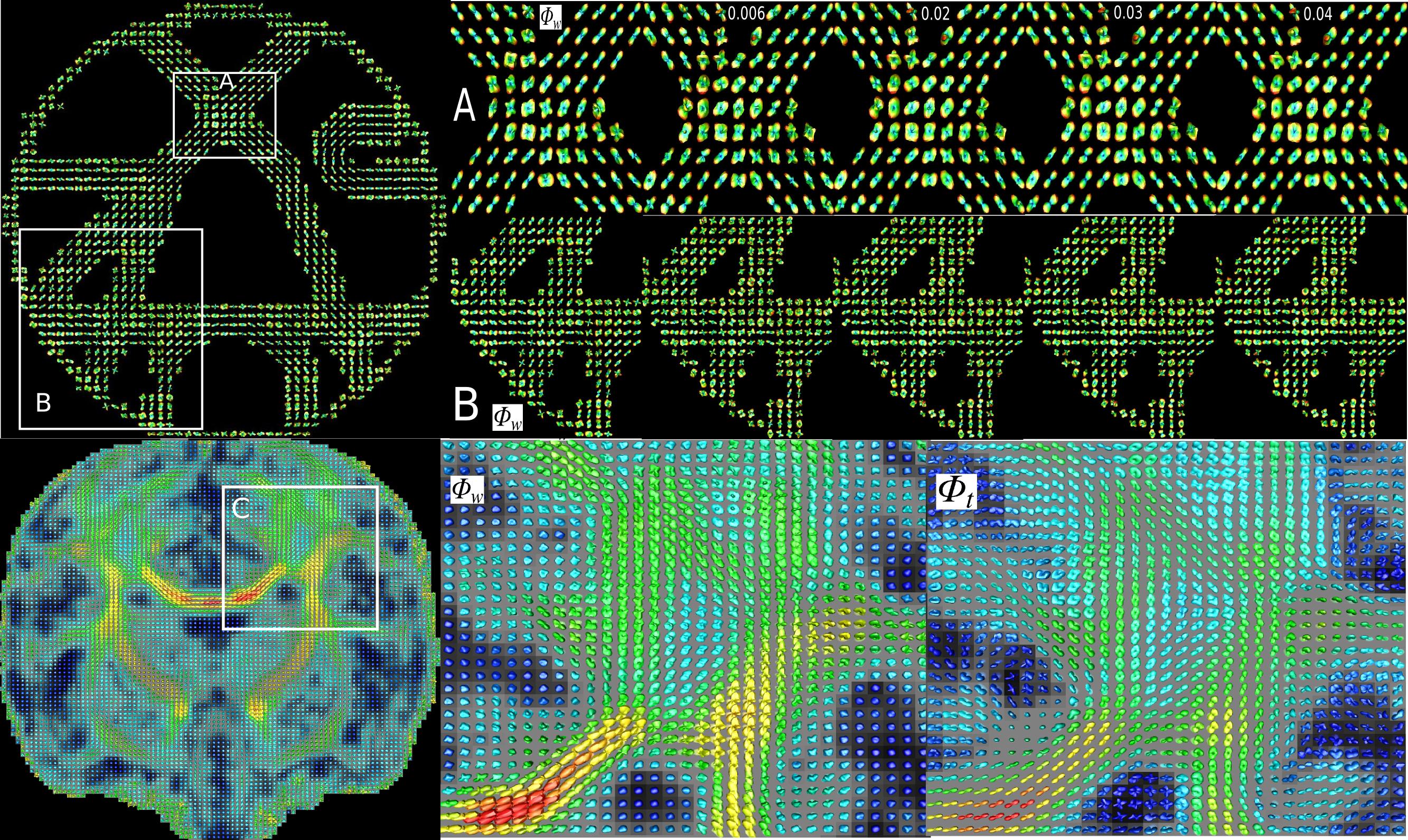
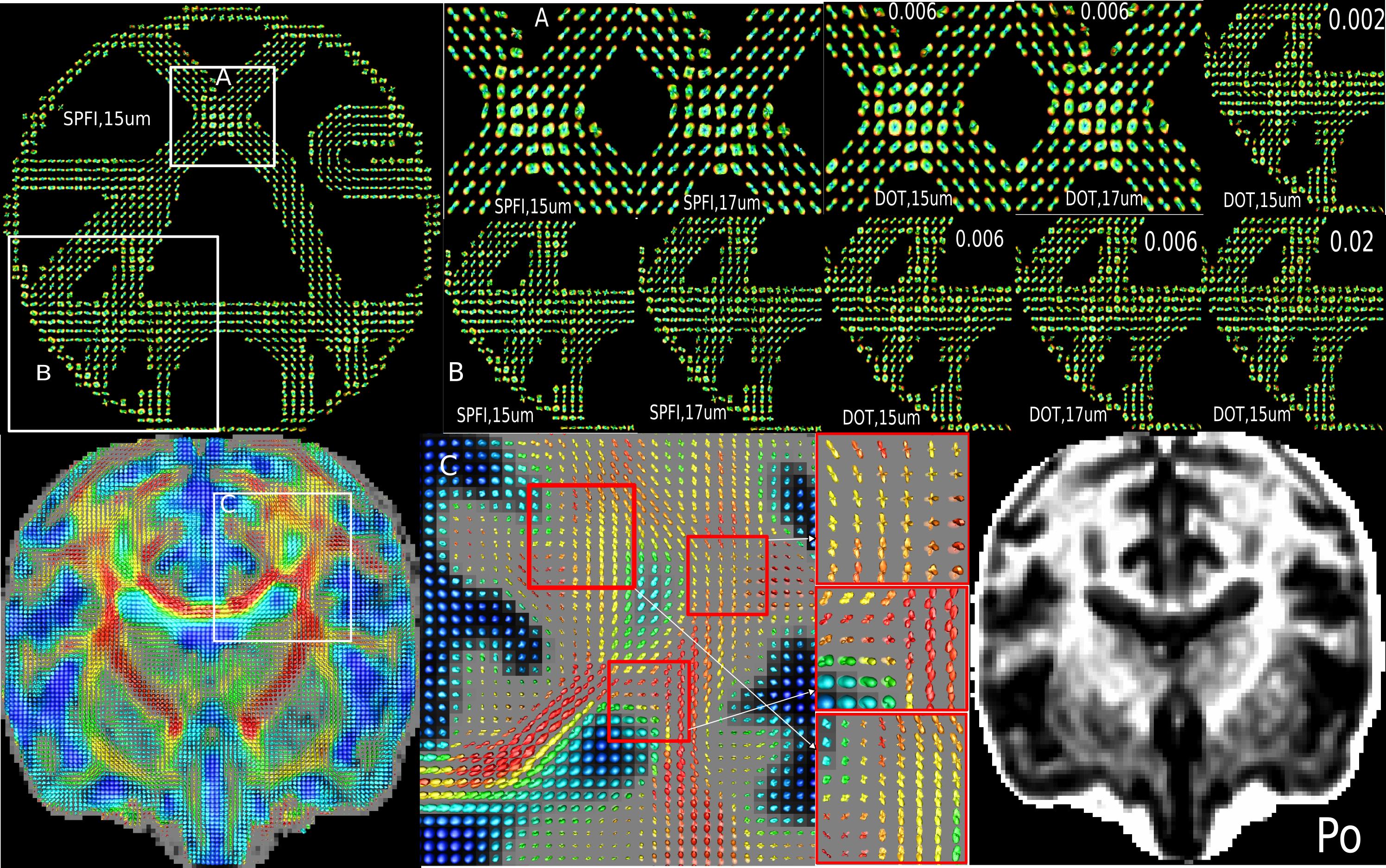
In diffusion MRI field, Ensemble Average Propagator (EAP) has full information about the diffusion process which reflects the complex tissue micro-structure. Its radial integral, named Orientation Distribution Function (ODF), is the probability density function that is used to describe the probability of the fiber direction. They both play very important roles in dMRI data process. How to analytically and robustly estimate EAP and ODF are at the heart of dMRI research.

Diffusion Spectrum Imaging (DSI) is the classical method to estimate EAP via Numerical Fourier Transform. Once EAP is estimated, ODF could be approximated via numerical integral. However DSI needs a lot of samplings and very large b values. Many papers proposed how to estimate EAP and ODF from single shell HARDI data with some unreal assumptions. Recently several methods based on mono-exponential decay assumption (MEDA) were proposed for single shell data. Although MEDA has been extended to multi-exponential model so that it can reduce the modeling error and work for the data from multiple shells, it is impractical because a nonlinear fitting is needed for every direction, suffering from limited samples, local minima, computational complexity.

Recently Spherical Polar Fourier Imaging (SPFI) was proposed to estimate some EAP features from multiple shell data without any assumption. However, the original SPFI proposed by Dr. Assemlal was a numerical solution using Parseval's theorem. It could be called as nSPFI. Here we present a novel analytical solution for SPFI. We call it aSPFI.

In aSPFI, we propose two linear transformations. One is from the q-space signal to the EAP profile. The other one is from the q-space signal to the ODF. They are analytical and linear, which makes them extremely fast. aSPFI naturally combines the DWI signals with different b-values. It works well especially for the data with low SNR, low anisotropy, and non-exponential decay.

Publications on this topic include:

up